Exercise 1: Acetic Acid

Hirshfeld surfaces, hydrogen bonding and electrostatics

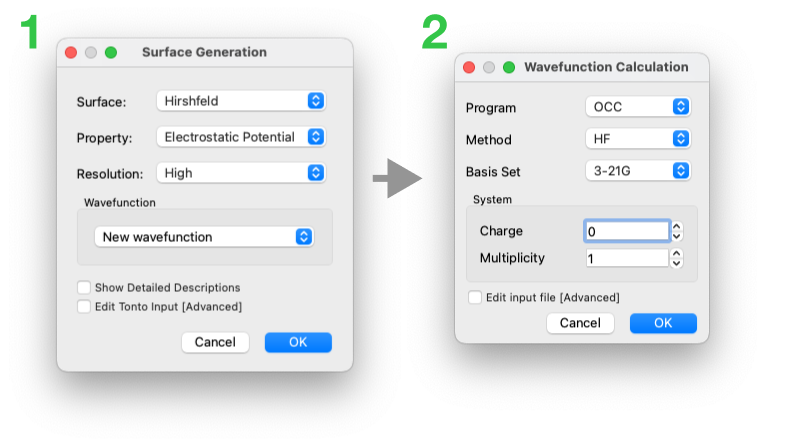
Initial Surface Generation

1. Open the ACETAC07 CIF , select the molecule and generate the Hirshfeld surface (HS) 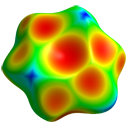, with a HF/3-21G electrostatic potential (using Tonto) mapped on the surface.

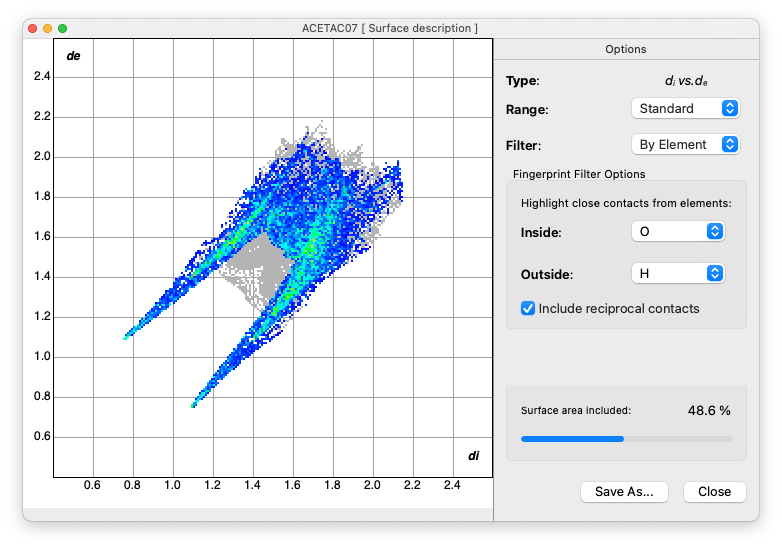
Fingerprint Plot Analysis

2. Generate the 'Fingerprint Plot' and explore its characteristics:
   • Use the Filter option to identify regions associated with specific atom···atom contacts (e.g. O···H and H···O separately)
   • Examine the patterns and features in the plot

Surface Properties

3. On the same surface, view some the default properties mapped on the same surface:
   • $d_i$
   • $d_e$
   • $d_\text{norm}$
   • 'Fragment Patch'
   • 'Shape Index'
   • 'Curvedness'

tip

Cycle through these one by one using the Surface dialog at bottom right of the graphics window. For each property, note the minimum/maximum values provided. You can also access surface area and volume for this HS this way.

Fragment Patch Analysis

4. The 'fragment patch' property is useful for identifying how many molecules (actually other surfaces) are in contact with the HS (effectively the first coordination sphere):
   • Click on the Information icon to reveal:
     • Details on various surface properties
     • Summary of atom···atom breakdowns for the fingerprint plot
     • Areas of each fragment patch
     • Additional analytical information

Electrostatic Potential Analysis

5. Return to the electrostatic potential surface and:
   • Rescale the surface property to limit the range to ±0.025 au
   • This highlights regions of:
     • Strong electronegative (red) character
     • Strong electropositive (blue) character

note

Enable surface transparency to better see the molecular structure beneath the surface

Interaction Analysis

6. To identify interactions:
   • Right-click on specific faces of the HS
   • Select Generate External Fragment to examine the interaction with the carboxylic acid group
   • Note: This structure shows hydrogen bonded chains (catemer motif) rather than the typical cyclic dimers found in many carboxylic acids
   • Use Clone Surface to create a chain of molecules/surfaces linked in this manner:

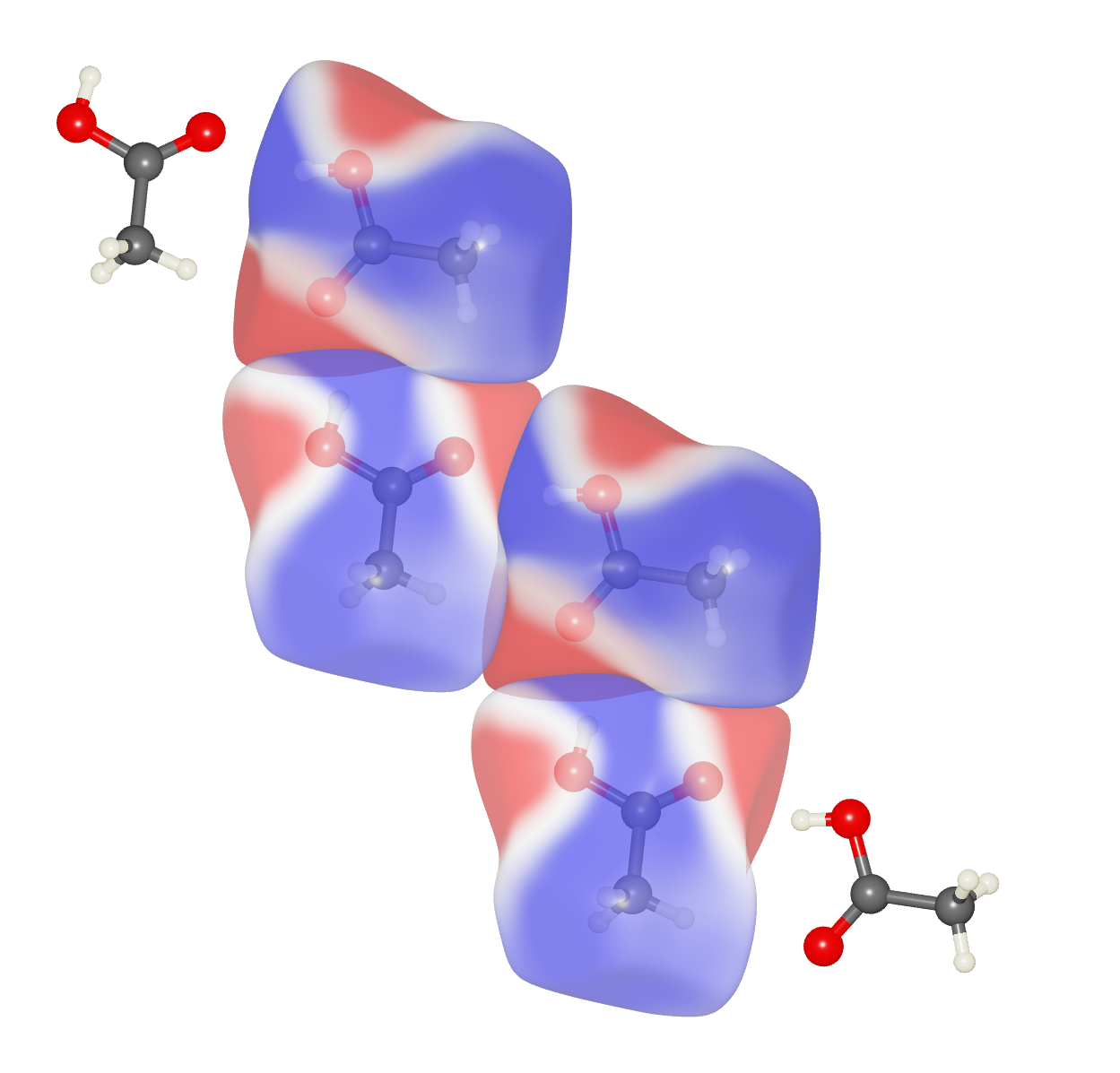
Interesting Observation

Look for the strong pattern of electrostatic complementarity between adjacent hydrogen-bonded molecules (red regions in one molecule adjacent to blue regions of its neighbour, and vice versa).

Surface Management

7. Managing surfaces:
   • Deselect individual surfaces by clicking on the ticks beside the surfaces
   • Note the hierarchy of surfaces:
     • Parent surface
     • Clones (related by specified symmetry operations)
   • Deselect the parent surface to remove all surfaces
   • Use the Display menu to show Hydrogen Bonds

Energy Calculations

8. For energy analysis:
   • Right click on the graphics window background
   • Select Reset Crystal to return to a single molecule
   • Use Show/Hide contact atoms to:
     • Generate neighbouring atoms
     • Select the hydrogen-bonded molecule
     • Remove remaining contact atoms
9. Calculate interaction energies:
   • Select one or both molecules
   • Click Calculate Energies
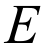
   • Select Energies from user-defined wavefunction
   • Expected result: -33.8 kJ/mol
   • Examine the energy breakdown in the Information dialog:
     • Electrostatic component
     • Dispersion component
     • Other energy terms

caution

Consider whether these energies are meaningful to within 1 kJ/mol

Cluster Analysis

10. For analyzing multiple molecular pairs:
    • Select a molecule
    • Use Generate Atoms within Radius (default 3.8 Å)
    • Complete all fragments
    • Calculate energies for all 7 unique molecular pairs
    • Note the color-coding in the graphics window

Energy Frameworks

11. Create energy frameworks:
    • Select Display / Energy frameworks
    • Examine different energy diagrams:
      • Coulomb Energy ($E_\text{ele}$)
      • Dispersion Energy ($E_\text{dis}$)
      • Total Energy ($E_\text{tot}$)
    • Use Show Options to customize the display

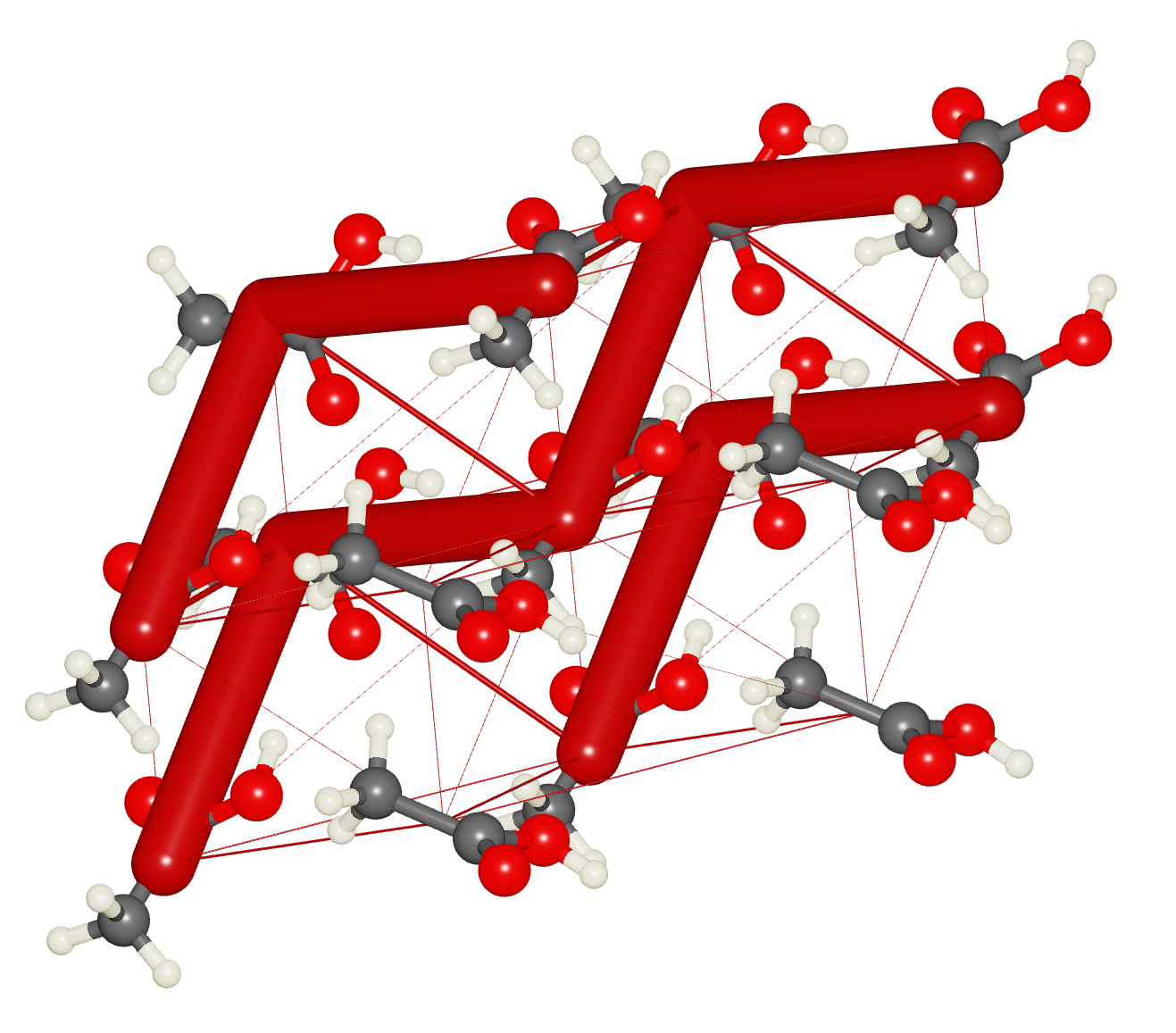
Lattice Energy Calculation

12. Calculate lattice energy:
    • Use the Information / Energies window
    • Multiply the $N$ and $E_\text{tot}$ columns (vector product)
    • Divide by 2
    • For 3.8 Å cluster: -62.6 kJ/mol
    • For 8 Å cluster (32 unique pairs): -70.4 kJ/mol

Comparison with Experimental Data

Compare your calculated results with the experimental sublimation enthalpy (~70±1 kJ/mol). The agreement is surprisingly good and will be explored further in Exercise 6.